Close Search Advanced
Journals
Resources
About Us
Open Access

Convergence of an Embedded Exponential-Type Low-Regularity Integrators for the KdV Equation Without Loss of Regularity

Convergence of an Embedded Exponential-Type Low-Regularity Integrators for the KdV Equation Without Loss of Regularity
Preview
Add to basket

Year:    2021

Author:    Yongsheng Li, Yifei Wu, Fangyan Yao

Annals of Applied Mathematics, Vol. 37 (2021), Iss. 1 : pp. 1–21

Abstract

In this paper, we study the convergence rate of an Embedded exponential-type low-regularity integrator (ELRI) for  the  Korteweg-de Vries equation. We develop some new harmonic analysis techniques to handle the "stability" issue. In particular, we use a new stability estimate which allows us to avoid the use of the fractional Leibniz inequality,

image.png

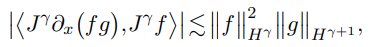
and replace it by suitable inequalities without loss of regularity. Based on these techniques, we prove that the ELRI scheme proposed in [41] provides $\frac12$-order convergence accuracy  in $H^\gamma$ for any initial data belonging to $H^\gamma$ with $\gamma >\frac32$, which  does not require any additional derivative assumptions.

Submit Article

You do not have full access to this article.

Already a Subscriber? Sign in as an individual or via your institution

Journal Article Details

Publisher Name:    Global Science Press

Language:    English

DOI:    https://doi.org/10.4208/aam.OA-2020-0001

Annals of Applied Mathematics, Vol. 37 (2021), Iss. 1 : pp. 1–21

Published online:    2021-01

AMS Subject Headings:    Global Science Press

Copyright:    COPYRIGHT: © Global Science Press

Pages:    21

Keywords:    The KdV equation numerical solution convergence analysis error estimate low regularity fast Fourier transform.

Author Details

Yongsheng Li

Yifei Wu

Fangyan Yao

  1. Quantifying and eliminating the time delay in stabilization exponential time differencing Runge–Kutta schemes for the Allen–Cahn equation

    Zhang, Hong | Liu, Lele | Qian, Xu | Song, Songhe

    ESAIM: Mathematical Modelling and Numerical Analysis, Vol. 58 (2024), Iss. 1 P.191

    https://doi.org/10.1051/m2an/2023101 [Citations: 2]

  2. Embedded exponential-type low-regularity integrators for KdV equation under rough data

    Wu, Yifei | Zhao, Xiaofei

    BIT Numerical Mathematics, Vol. 62 (2022), Iss. 3 P.1049

    https://doi.org/10.1007/s10543-021-00895-8 [Citations: 14]

  3. Low regularity exponential-type integrators for the “good” Boussinesq equation

    Li, Hang | Su, Chunmei

    IMA Journal of Numerical Analysis, Vol. 43 (2023), Iss. 6 P.3656

    https://doi.org/10.1093/imanum/drac081 [Citations: 2]

  4. Time splitting method for nonlinear Schrödinger equation with rough initial data in L2

    Choi, Hyung Jun | Kim, Seonghak | Koh, Youngwoo

    Journal of Differential Equations, Vol. 417 (2025), Iss. P.164

    https://doi.org/10.1016/j.jde.2024.11.018 [Citations: 0]

  5. A second-order low-regularity correction of Lie splitting for the semilinear Klein–Gordon equation

    Li, Buyang | Schratz, Katharina | Zivcovich, Franco

    ESAIM: Mathematical Modelling and Numerical Analysis, Vol. 57 (2023), Iss. 2 P.899

    https://doi.org/10.1051/m2an/2022096 [Citations: 1]

  6. A constructive low-regularity integrator for the one-dimensional cubic nonlinear Schrödinger equation under Neumann boundary condition

    Bai, Genming | Li, Buyang | Wu, Yifei

    IMA Journal of Numerical Analysis, Vol. 43 (2023), Iss. 6 P.3243

    https://doi.org/10.1093/imanum/drac075 [Citations: 3]

  7. An Embedded Exponential-Type Low-Regularity Integrator for mKdV Equation

    Ning, Cui | Wu, Yifei | Zhao, Xiaofei

    SIAM Journal on Numerical Analysis, Vol. 60 (2022), Iss. 3 P.999

    https://doi.org/10.1137/21M1408166 [Citations: 8]

  8. Gauge-Transformed Exponential Integrator for Generalized KdV Equations with Rough Data

    Li, Bing | Wu, Yifei | Zhao, Xiaofei

    SIAM Journal on Numerical Analysis, Vol. 61 (2023), Iss. 4 P.1689

    https://doi.org/10.1137/22M1530501 [Citations: 2]

  9. The BDF3/EP3 Scheme for MBE with No Slope Selection is Stable

    Li, Dong | Quan, Chaoyu | Yang, Wen

    Journal of Scientific Computing, Vol. 89 (2021), Iss. 2

    https://doi.org/10.1007/s10915-021-01642-5 [Citations: 4]

  10. The Operator-Splitting Method for Cahn-Hilliard is Stable

    Li, Dong | Quan, Chaoyu

    Journal of Scientific Computing, Vol. 90 (2022), Iss. 1

    https://doi.org/10.1007/s10915-021-01740-4 [Citations: 8]

  11. A first-order Fourier integrator for the nonlinear Schrödinger equation on 𝕋 without loss of regularity

    Wu, Yifei | Yao, Fangyan

    Mathematics of Computation, Vol. (2021), Iss.

    https://doi.org/10.1090/mcom/3705 [Citations: 6]