Year: 2021
Author: Maochun Zhu, Yifeng Zheng
Journal of Partial Differential Equations, Vol. 34 (2021), Iss. 2 : pp. 116–128
Abstract
In this paper, we are concerned with a sharp fractional Trudinger-Moser type inequality in bounded intervals of R under the Lorentz-Sobolev norms constraint. For any $1<q<∞$ and $β≤ \big(\sqrt{π} \big)^{q'} \equiv β_q, q'= \frac{q}{q-1}$, we obtain
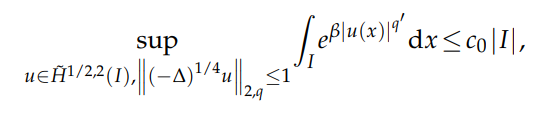
and $β_q$ is optimal in the sense that
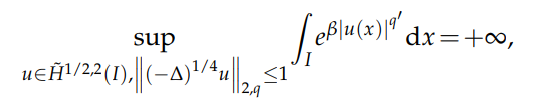
for any $β>β_q$. Furthermore, when $q$ is even, we obtain
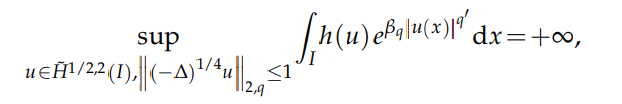
for any function $h : [0,∞)→[0,∞)$ with lim$_{t→∞} h(t) = ∞$. As for the key tools of proof, we use Green functions for fractional Laplace operators and the rearrangement of a convolution to the rearrangement of the convoluted functions.
You do not have full access to this article.
Already a Subscriber? Sign in as an individual or via your institution
Journal Article Details
Publisher Name: Global Science Press
Language: English
DOI: https://doi.org/10.4208/jpde.v34.n2.2
Journal of Partial Differential Equations, Vol. 34 (2021), Iss. 2 : pp. 116–128
Published online: 2021-01
AMS Subject Headings:
Copyright: COPYRIGHT: © Global Science Press
Pages: 13
Keywords: Trudinger-Moser inequality Lorentz-Sobolev space bounded intervals.

