Year: 2019
Author: Daomin Cao, Zhongyuan Liu
Annals of Applied Mathematics, Vol. 35 (2019), Iss. 3 : pp. 221–249
Abstract
In this paper, we construct stationary classical solutions of the shallow
water equation with vanishing Froude number Fr in the so-called lake model.
To this end we need to study solutions to the following semilinear elliptic
problem
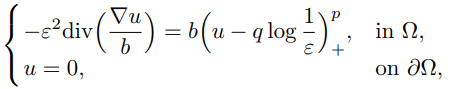
for small ε > 0, where p > 1, div(\frac{∇q}{b})
= 0 and Ω ⊂ \mathbb{R}2 is a smooth bounded
domain.
We show that if \frac{q^2}{b} has m strictly local minimum (maximum) points \widetilde{z}_i, i =
1, · · · , m, then there is a stationary classical solution approximating stationary m points vortex solution of shallow water equations with vorticity \sum\limits_{i=1}^m \frac{2πq(\widetilde{z}_i)}{b(\widetilde{z}_i)}.
Moreover, strictly local minimum points of \frac{q^2}{b} on the boundary can also give
vortex solutions for the shallow water equation.
You do not have full access to this article.
Already a Subscriber? Sign in as an individual or via your institution
Journal Article Details
Publisher Name: Global Science Press
Language: English
DOI: https://doi.org/2019-AAM-18081
Annals of Applied Mathematics, Vol. 35 (2019), Iss. 3 : pp. 221–249
Published online: 2019-01
AMS Subject Headings: Global Science Press
Copyright: COPYRIGHT: © Global Science Press
Pages: 29
Keywords: shallow water equation free boundary stream function vortex solution.

