Year: 2020
Author: Stan Alama, Lia Bronsard, Petru Mironescu
Analysis in Theory and Applications, Vol. 36 (2020), Iss. 2 : pp. 128–160
Abstract
We consider minimizers of the energy
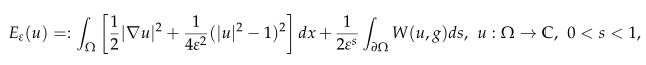
in a two-dimensional domain $\Omega$, with weak anchoring potential
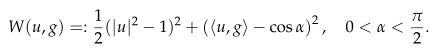
This functional was previously derived as a thin-film limit of the Landau-de Gennes energy, assuming weak anchoring on the boundary favoring a nematic director lying along a cone of fixed aperture, centered at the normal vector to the boundary.
In the regime where $s [\alpha^2+(\pi-\alpha)^2]<\pi^2/2$, any limiting map $u_\ast:\Omega\to{\mathbb S}^1$ has only boundary vortices, where its phase jumps by either $2\alpha$ (light boojums) or $2(\pi-\alpha)$ (heavy boojums). Our main result is the fine-scale description of the light boojums.
Journal Article Details
Publisher Name: Global Science Press
Language: English
DOI: https://doi.org/10.4208/ata.OA-0020
Analysis in Theory and Applications, Vol. 36 (2020), Iss. 2 : pp. 128–160
Published online: 2020-01
AMS Subject Headings: Global Science Press
Copyright: COPYRIGHT: © Global Science Press
Pages: 33
Keywords: Nematics thin-film limit Ginzburg-Landau type energy weak anchoring boundary vortices asymptotic profile.
Author Details
-
On minimizers of the 2D Ginzburg–Landau energy with tangential anchoring
Alama, Stan
Bronsard, Lia
van Brussel, Lee
Nonlinear Analysis, Vol. 232 (2023), Iss. P.113276
https://doi.org/10.1016/j.na.2023.113276 [Citations: 1]

